1804 Practice problems exam 2, Spring 18 Solutions Problem 1 Harmonic functions (a) Show u(x;y) = x3 3xy2 3x2 3y2 is harmonic and nd a harmonic conjugate It's easy to compute u x= 3x2 3y2 6x;Using the above identity taking a = x − y, b = y − z and c = z − x, we have a b c = x − y y − z z − x = 0 then the equation (x − y) 3 (y − z) 3 (z − x) 3 can be factorised as followsFind the Maclaurin series expansion for f = sin(x)/x The default truncation order is 6 T = x^5/1 x^3/6 x y^4/24 y^2/2 z^5/1 z^4/24 z^3/6 z^2/2 z 2 You can use the sympref function to modify the output order of a symbolic polynomial Redisplay the polynomial in ascending order

The 1 3 Km Road Expansion Cost Near Ap Cm Ys Jagan House Is 5 Crores Social News Xyz
What is the formula of (x+y+z)^3
What is the formula of (x+y+z)^3- (xy)^3 (yz)3 (zx)^3 = 3(xy)(yz)(zx) That is it no constraints etc It mentions "This can be done by expanding out the brackets, but there is a more elegant solution" Homework Equations The Attempt at a Solution First of all this only seems to hold in special cases as I have substituted random values for x,y and z and they do not agree⋅(x)3−k ⋅(y)k ∑ k = 0 3
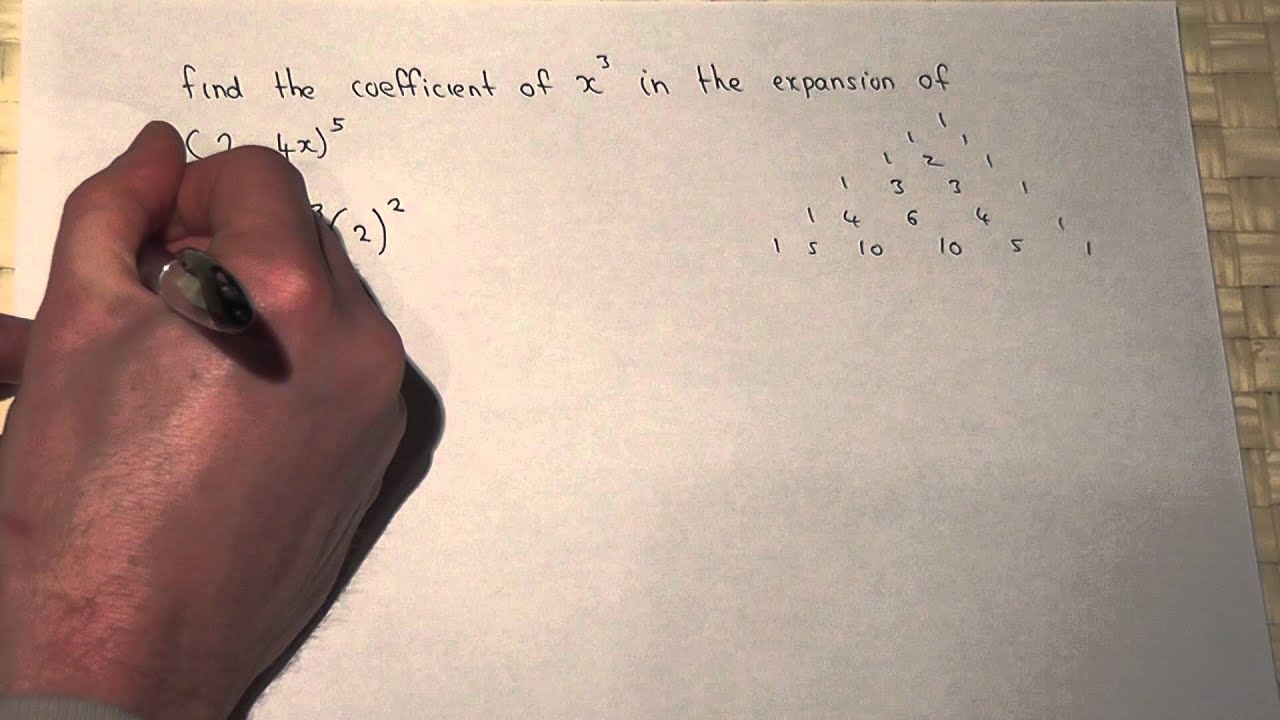



Binomial Theorem Finding The Coefficient Of X 3 In 2 4x 5 Youtube
Using Properties of Determinants, Prove that (2y,Yzx,2y),(2z,2z,Zxy),(Xyz,2x,2x)=(XYZ)^3 CBSE CBSE (Arts) Class 12 Question Papers 17 Textbook Solutions Important Solutions 24 Question Bank Solutions Concept Notes & Videos 532 Time Tables 1811 Factoring x 3 y 3 z 3 Theory A sum of two perfect cubes, a 3 b 3 can be factored into (ab) • (a 2abb 2) Proof (ab) • (a 2abb 2) = a 3a 2 b ab 2 ba 2b 2 a b 3 = a 3 (a 2 bba 2)(ab 2b 2 a) b 3 = a 3 0 0 b 3 = a 3 b 3 Check x 3 is the cube of x 13 Answers3 For the nontrivial interpretation, you're looking for nonnegative solutions of a b c = n (each of these corresponds to a term x a y b z c ) Code each of these solutions as 1 a 0 1 b 0 1 c, for example ( 2, 3, 5) would be coded as Now it should be easy to see why the answer is ( n 2 n)
We obtain f (a)=c0 Next, we takeWe will see that for the expansion of a trinomial $(x y z)^n$, an analogous theorem holds For example, suppose that we want to expand the trinomial $(x y z)^3$ We will have there be $\binom{3 3 1}{3} = \binom{5}{3} = 10$ nonnegative integer solutions to this equationFind the sumofproducts expansion of the Boolean function F(w;x;y;z) that has the value 1 if and only if an odd number of w;x;y, and z have the value 1 Need to produce all the minterms that have an odd number of 1s The DNF is simply, wxyz wxyz wxyz wxyz wx yz wxy z wxy z wx y z
U yy= 6x 6 It's clear that r2u= u xx u yy= 0, so uis harmonic If vis a conjugate harmonic function to u, then uivis analytic and the CauchyRiemann Ex 42, 9 By using properties of determinants, show that 8 (x&x2&yz@y&y2&zx@z&z2&xy) = (x – y) (y – z) (z – x) (xy yz zx) Solving LHS 8 (𝑥&𝑥^2&𝑦𝑧@𝑦&𝑦^2&𝑧𝑥@𝑧&𝑧^2&𝑥𝑦) Applying R1→ R1 – R2 = 8 (𝑥−𝑦&𝑥^2−𝑦^2&𝑦𝑧−𝑥𝑧@𝑦&𝑦^2&𝑧𝑥@𝑧&𝑧^2&𝑥𝑦) ExTo ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Verify that `x^3y^3z^33x y z=1/2(xyz)(xy)^2(yz)^2(zx)^2`
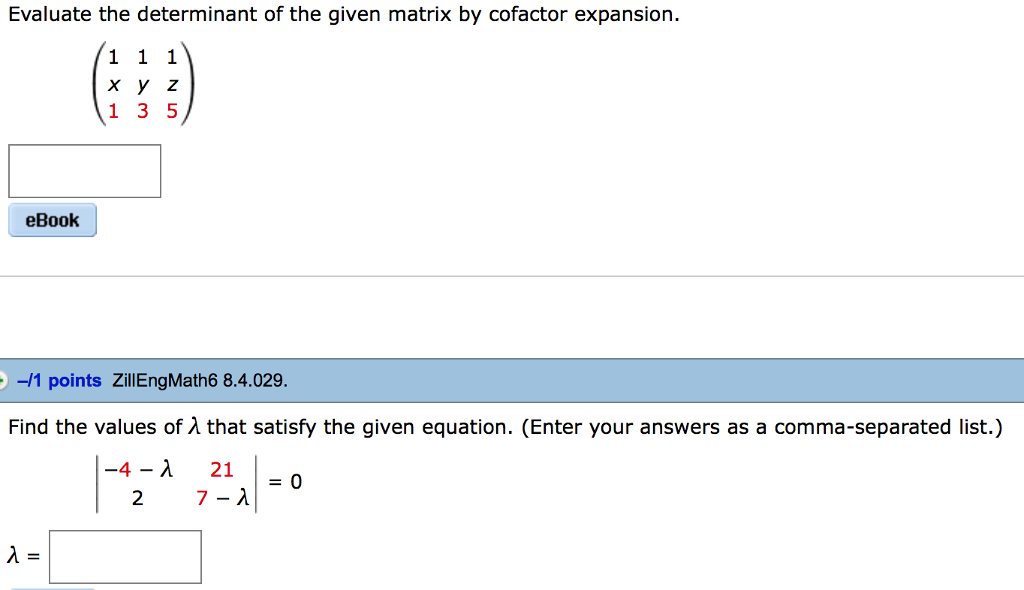



Evaluate The Determinant Of The Given Matrix By Chegg Com




How To Simplify Math X Y Z 2 X Y Z 2 X Y Z 2 X Y Z 2 Math Quora
I is x i or x i 3 A sumofproducts expansion or disjunctive normal form of a Boolean function is the function written as a sum of minterms Discussion Consider a particular element, say (0,0,1), in the Cartesian product There is a unique Boolean product that uses each of the variables x, y, z or its complement (but not both) and hasCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyEvaluate the following java expression,if x=3,y=5 and z=10 zyyzx Posted on by Views Score Share EngineeringCS EngineeringIS mca JIT Davangere SEMVI Java



Solved 5 Points Find The Output Of The Given Circuit X X Y 2 5 Points Find The Sum Of Products Expansion For The Function F X Y Z Given I Course Hero




Top 10 Strongest Rank 10 Xyz Monsters In Yu Gi Oh Hobbylark
X 0 0 = 3 {substituted 0 for y and z} x = 3 {combined like terms} coordinates are (3, 0, 0) Algebra 3 Section 35 Systems with Three Variables The graph of an equation in three variables, such as, Ax By Cz = D where A,B, and C are not all zero, is a plane yintercept x y z = 3 {the equation} 0 y 0 = 3 {substituted 0 for x and zExpand (xy)^3 (x y)3 ( x y) 3 Use the Binomial Theorem x3 3x2y3xy2 y3 x 3 3 x 2 y 3 x y 2 y 3Start your free trial In partnership with You are being redirected to Course Hero I want to submit the same problem to Course Hero Cancel




Hec Ras Results
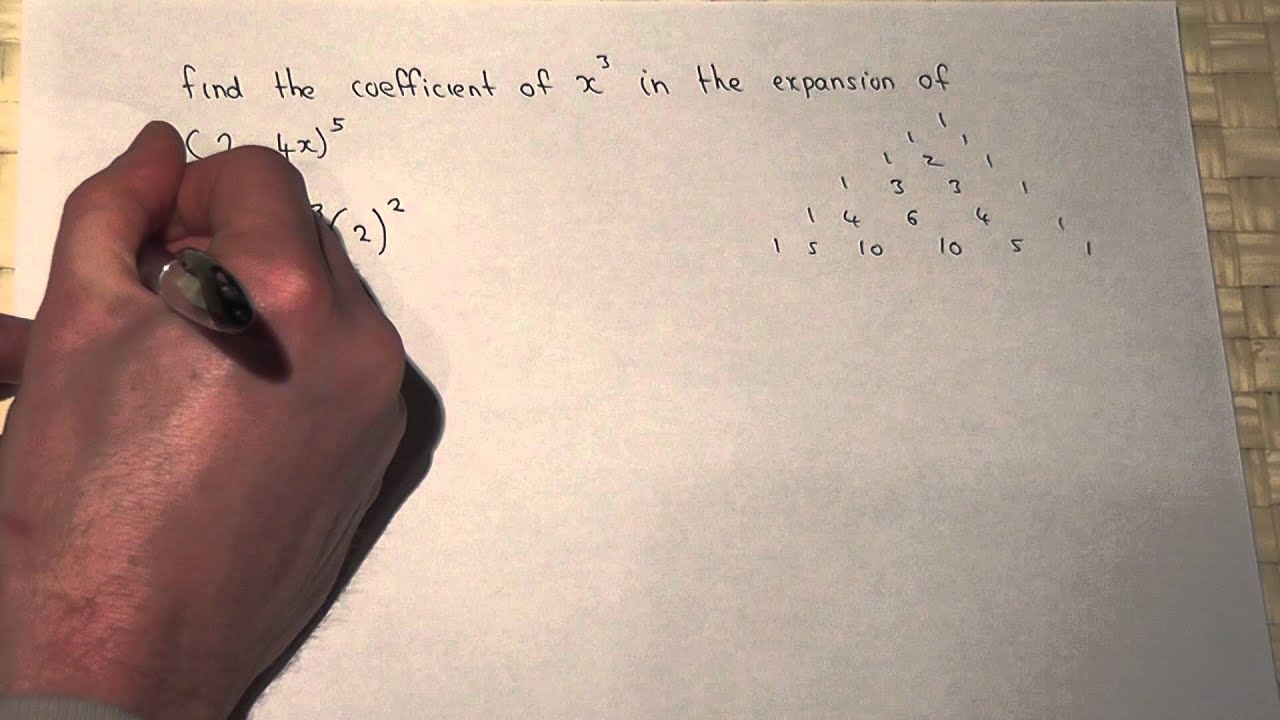



Binomial Theorem Finding The Coefficient Of X 3 In 2 4x 5 Youtube
Explanation (x −y)3 = (x − y)(x −y)(x −y) Expand the first two brackets (x −y)(x − y) = x2 −xy −xy y2 ⇒ x2 y2 − 2xy Multiply the result by the last two brackets (x2 y2 −2xy)(x − y) = x3 − x2y xy2 − y3 −2x2y 2xy2 ⇒ x3 −y3 − 3x2y 3xy2 Always expand each term in the bracket by all the otherLaurent Expansion Let f (z) = z(z − 1) −1 If we choose to make the Laurent expansion about z 0 = 0, then r > 0 and R < 1 These limitations arise because f (z) diverges both at z = 0 and z = 1 A partial fraction expansion, followed by the binomial expansion of (1 − zThe following are algebraix expansion formulae of selected polynomials Square of summation (x y) 2 = x 2 2xy y 2 Square of difference (x y) 2 = x 2 2xy y 2 Difference of squares x 2 y 2 = (x y) (x y) Cube of summation (x y) 3 = x 3 3x 2 y 3xy 2 y 3 Summation of two cubes x 3 y 3 = (x y) (x 2 xy y 2) Cube




Chapter 4 Expansion R K Bansal Solutions For Class 6 Mathematics Maharashtra Topperlearning




X Y Z 3 Expand Novocom Top
(x y) 3 = x 3 3x 2 y 3xy 2 y 3 Example (1 a 2 ) 3 = 1 3 31 2 a 2 31(a 2 ) 2 (a 2 ) 3 = 1 3a 2 3a 4 a 6 (x y z) 2 = x 2 y 2 z 2 2xy 2xz 2yzFree expand & simplify calculator Expand and simplify equations stepbystepThe binomial theorem (or binomial expansion) is a result of expanding the powers of binomials or sums of two terms The coefficients of the terms in the expansion are the binomial coefficients ( n k) \binom {n} {k} (kn ) The theorem and its generalizations can be used to prove results and solve problems in combinatorics, algebra, calculus



Search Q Expanding Polynomials Tbm Isch
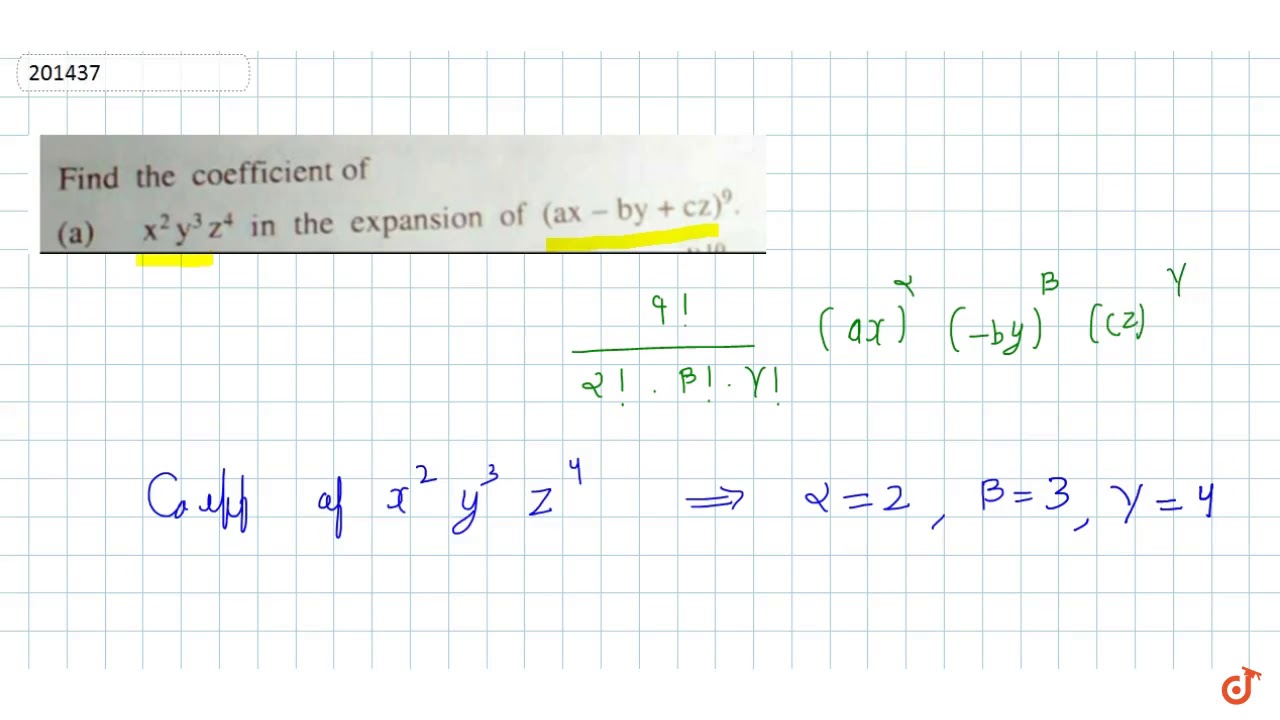



Find The Coefficient Of X 2 Y 3 Z 4 In The Expansion Of Ax By Cz 9 Youtube



0 件のコメント:
コメントを投稿