Differential Equation Calculator The calculator will find the solution of the given ODE firstorder, secondorder, nthorder, separable, linear, exact, Bernoulli, homogeneous, or inhomogeneous Initial conditions are also supported Your input solve $$$ y ' \left (x \right) = x^ {2} $$$(c) y00 xy2y0 −y3 = exy is a nonlinear equation; In this section we introduce the method of undetermined coefficients to find particular solutions to nonhomogeneous differential equation We work a wide variety of examples illustrating the many guidelines for making the initial guess of the form of the particular solution that is needed for the method

Deber No 9 Solucion En Serie De Potencias En Torno A Puntos Singulares Ensenanza De Matematica
(1-x^2)y''-2xy'+6y=0
(1-x^2)y''-2xy'+6y=0-Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history1 dy 2y = 0 dx Definimos el factor integrante p (x) = 2 ´ factor integrante e 2dx = e2x multiplicamos la ecuacion por el factor integrante dy e2x dx 2e2x = 0 el lado izquierdo de la ecuacion se reduce a d 2x dx e y =0 separamos variables e integramos ´ d 2x ´ dx e y =0 dx c e2x y = c y = ce−2x 2 dy = 3y dx forma lineal dy




Engineering Mathematics Notes
Sección 61 Reposo de las series de potencias;29 Show that y1 = x2 and y2 = x3 are two different solu tions of x2 y"4xy' 6y = 0, both satisfying the initial conditions y (0) = 0 = y' (0) Explain why these facts do not contradict Theorem 2 (with respect to the guaranteed uniqueness) 30 (a) Show that Yl = x3 and yz = lx3 1 are linearly inde Q727 27 Find a power series in \(x\) for the general solution of \(1x^2)y''4xy'2y=0 \tag{A}\ Use (a) and the formula \{1\over1
En otras palabras, cuando x está en el intervalo de6C3 For the ODE y 2xy 2y = 0, a) find two independent series solutions y1 and y2;Zill Differential Equations 9e Chapter 1 Form B 13 Determine a region of the xy−plane for which the given differential equation has a unique solution passing through a point (x0,y0) in the region (x −1)2(y 2)y′ = 1 14
Question Find The Solution Of Legendre's DE(1x^2)y''2xy'6y=0 Give The Solution Asy=c0all The The Termsc13 Tarms Here This problem has been solved!1 B ` AI T ˆ A P PHU O NG TR ` INH VI PH ˆ AN 1) 2xy y" = y 2 − 1 HD gia ' i y = p 2xpp = p 2 − 1 x(p 2 − 1) = 0 2pdp p 2 − 123doc thư viện trực tuyến, download tài liệu, tải tài liệu, sách, sách số, ebook, audio book, sách nói hàng1 x2 Multiplying through by µ leads to the equation (3 y x2)dx(y − 1 x)dy = 0, which is exact There exists a function F which satisfies ∂F ∂x = 3 y x2, ∂F ∂y = y − 1 x (1) The first of these implies F(x,y) = 3x− y x g(y) Differentiating with respect to y and comparing the result with the second equation in (1) leads to



Http Www Iitg Ac In Jiten Lectures Lec15 Pdf




Math Infinity Differential Calculus Facebook
(b) 4x2y′′ (2x4 − 5x)y′ (3x2 2)y = 0 Solution (a) A0 = lim x→0 A(x) = lim x→0 xP(x) = lim x→0 cos2x−1 x2 = lim x→0 −2sin2x 2x = −2 B0 = lim x→0 B(x) = lim x→0 x2Q(x) = lim x→0 2 = 2 The indicial equation is r2 (A0(1) x2y00 2xy0 −2y =0; Use the method of undetermined coefficients to solve (1x 2 )y′′ – 2xy′ 6y = 0 Using the method of undetermined coefficients obtain a recursion formula relating the terms to the terms Give an explicit formula for for each and find the sum of the series Since this sum is equal to 0, we know that every coefficient of every power




Multiply X 2 4y 2 2xy 3x 6y 9 By X 2y 3
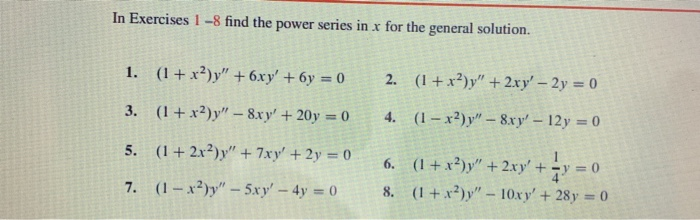



In Exercises 1 8 Find The Power Series In X For The Chegg Com
On the other hand, when the power series is convergent for all x, we say its radius of convergence is infinity,C) express the solution satisfying y(0) = 1, y (0) = −1 in terms of y1 and y2;Exam 1 Review Questions and Answers Part I Finding solutions of a given differential equation 1 Find the real numbers r such that y = ex is a solution of y00 −y0 − 30y = 0 Answer r = 6, −5



Http Www Math Uiuc Edu G Choi1 285finalreviewsol 03 Pdf



Http Www Tccollege Org Wp Content Uploads 19 08 Mathematics Mat4105 O D E M Sc Pdf
1 = x2 is a solution to the differential equation x2y00 2xy0 6y = 0, find a second linearly independent solution using the formula seen earlier Example Given that y 1 = x2 is a solution to the differential equation x2y00 2xy0 6y = 0, find the general solution to the differential equation using the substitution y 2(x) = u(x)y 1(x) 4 Hint Assume that a polynomial of the form y ( x) = x n a n − 1 x n − 1 ⋯ a 0 satisfies the ODE, and pug it in the ODE Then you shall find that n = 2 Next, you shall find that y = x 2 − 1 / 3 Observe that if y is a solution, then so is c y, for every c ∈ R Satisfaction of the condition y ( 1) = 2, implies that the soughtD) express the series in terms of elementary functions (ie, sum the series to an elementary function)




Differential Equations Class 12 Ncert Solutions




Calc Solutions Sec 2 5
Homework Statement Determine the IVP has bounded solution Legendre equation (1x^2)y''2xy'6y=0 ;This particular number ρ is called the r adiu s of c onv er ge nc e Remark 3 The number ρ is at least 0, as taking x = x0 gives P 0 which is clearly converging to 0;X (1 x 2y 2) = 0 , and (**) x = 0 or 1 x 2y 2 = 0 If x=0 in the original equation (x 2 y 2) 2 = 2x 22y 2, then (0y 2) 2 = 02y 2, y 4 2y 2 = 0 , y 2 ( y 2 1 ) = 0 , and y=0 Note, however, if x=0 and y=0 are substituted into Equation 1, we get the indeterminate form " 0/0 " Is y'=0 at the point (0, 0) , ie, does y'(0, 0) = 0




Solutions To Implicit Differentiation Problems




Assignment 1



0 件のコメント:
コメントを投稿